On Lusternik–Schnirelmann category and topological complexity of non-k-equal manifolds

Resumen

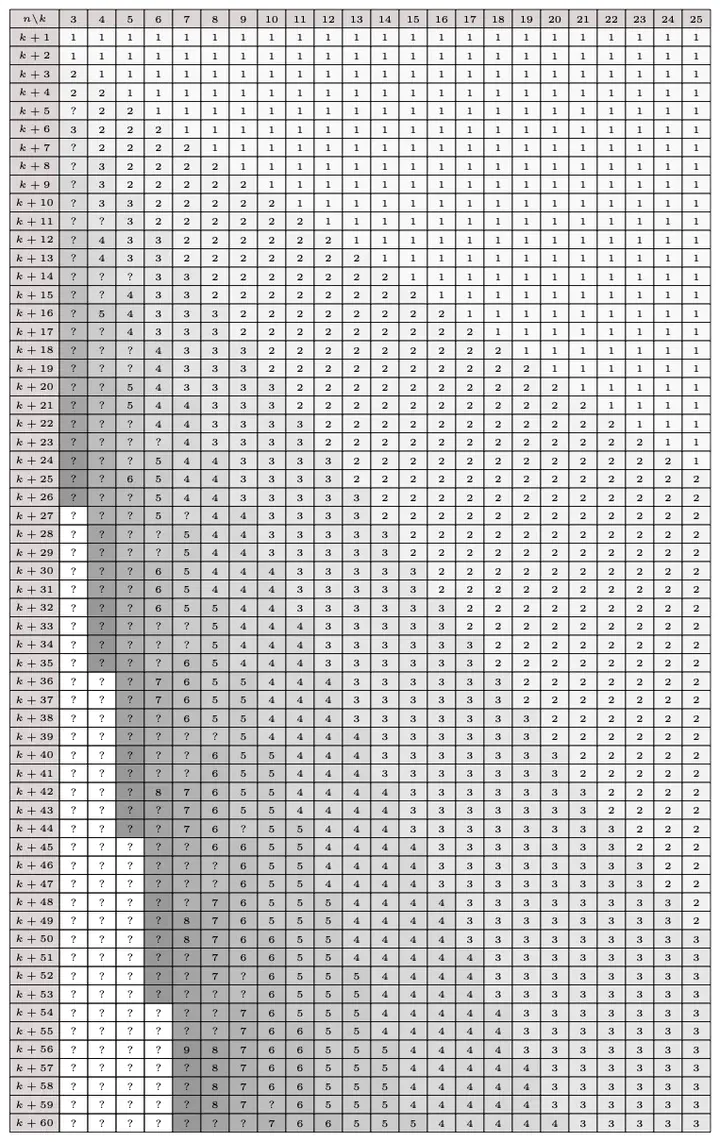
We compute the Lusternik-Schnirelmann category and all the higher topological complexities of non-$k$-equal manifolds $M_d^{(k)}(n)$ for certain values of $d$, $k$ and $n$. This includes instances where $M_d^{(k)}(n)$ is known to be rationally non-formal. The key ingredient in our computations is the knowledge of the cohomology ring $H^{\ast }(M_d^{(k)}(n))$ as described by Dobrinskaya and Turchin. A fine tuning comes from the use of obstruction theory techniques. The table shown above shows the Lusternik-Schnirelmann category values for the case $d=2$ and small values of $k$ and $n$.